P/W = 0.01
P/W = 1
P/W = ∞
3D Dome
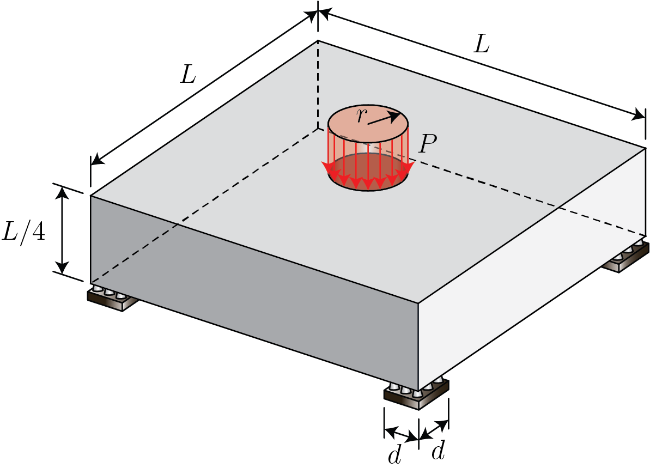
3D dome fixed at the four lower corners and subjected to a load of magnitude P applied at the center and distributed uniformly on a circle of prescribed radius. These results show the effects of self-weight on the optimization results for various values of P/W, in which W is the weight of the solid domain.
A unified approach for topology optimization with local stress constraints considering various failure criteria: von Mises, Drucker–Prager, Tresca, Mohr–Coulomb, Bresler–Pister and Willam–Warnke.
Problem description
The 3D model above corresponds to the stress-constrained topology optimization results for the box domain whose geometry is shown below. The optimization results were obtained using 250k regular hexahedral elements on a MATLAB implementation of an augmented Lagrangian-based formulation for topology optimization with local stress constraints based on the von Mises criterion.